Poster: Determining the Structural Stability of Lunar Lava Tubes.
Paper version
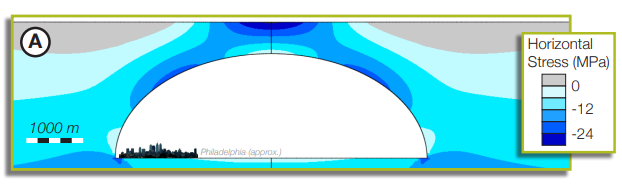
In Robert Heinlein’s short story, “The Menace from Earth”, the Lunar colony is depicted as using vast natural chambers under the Moon for air storage and as a personal flying venue. Heinlein’s chambers are now shown to be possible and, in fact, not the largest we might find under the Moon, as revealed in several papers at the 46th Lunar & Planetary Science Conference, March 16-20, 2015.